A Regularised Extended Ptychographical Iterative Engine for Low-dose and Fast Electron Ptychography

Session

EMAG - 4D STEM

Authors

Amirafshar Moshtaghpour (1, 2), Abner Velazco-Torrejon (1), Alex Robinson (2), Angus Kirkland (1, 4), Nigel Browning (2, 3, 5)

Affiliations

1. Rosalind Franklin Institute
2. University of Liverpool
3. Pacific Northwest National Lab
4. University of Oxford
5. Sivananthan Laboratories

Keywords

Ptychography, Four-dimensional Scanning Transmission Electron Microscopy, Subsampling

Abstract text

Four-dimensional Scanning Transmission Electron Microscopy (4D STEM) is a technique of collecting two-dimensional (2D) diffraction patterns for every position of a (focused or defocused) convergent electron beam [1]. Leveraging the redundancy in 4-D STEM data, electron Ptychography ̶̶ that is the recovery of a high-resolution electron-wave phase changes, referred here to as object phase ̶̶ is possible through a phase retrieval process using, e.g., Extended Ptychographical Iterative Engine (EPIE) [2], Difference Map (DM) [3], and Relaxed Averaged Alternating Reflections [4]. The success of phase recovery process requires, however, highly overlapping electron beams (typically more than 70% overlap ratio), which in turn amounts to a slow acquisition (~1ms dwell time for each probe position, or equivalently, diffraction pattern) and to an over-exposed sample. 

Subsampling probe positions has shown to be a promising dose-reduction solution to scanning (transmission) electron microscopy [5,6]. Similarly, an approach to achieve low-dose and fast 4D STEM is to subsample scanned probe positions, which results in an incomplete data with lower overlap ratio. Performing conventional electron Ptychography methods results in poor quality object phase images. An application of the theory of Compressive Sensing (CS) [7] showed that a complete set of 4D STEM measurements can be recovered from simulated incomplete data as if they were recorded by subsampling electron probe positions and the diffraction patterns [8], followed by performing a Wigner distribution deconvolution algorithm on the recovered 4D STEM data to obtain the object phase. 

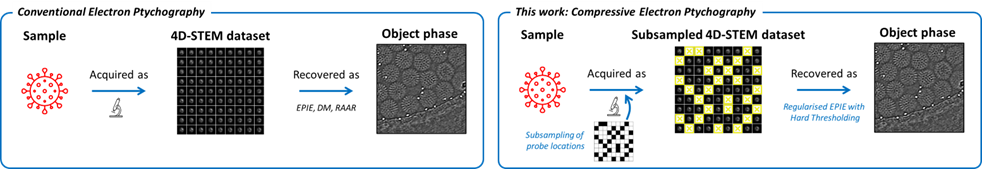
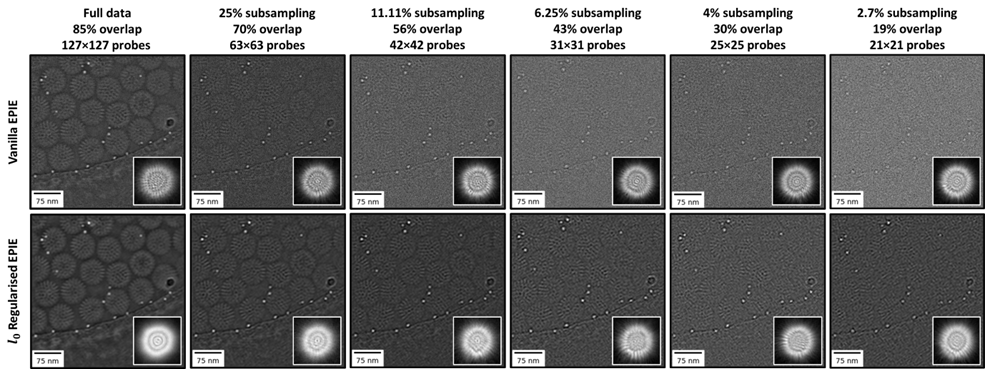
In this work, as illustrated in Fig. 1, we present a novel electron Ptychography algorithm that recovers the object phase directly from the subsampled 4D STEM data. Our approach is based on EPIE algorithm with a difference that an l₀ regularisation is performed every time the object image is updated. Intuitively, at every iteration, the algorithm distinguishes a natural object phase from arbitrary noise-like phase shifts according to their sparsity level in a transform domain.  In other word, l₀ regularisation enforces the object phase to have a sparse representation in, e.g., Fourier or Wavelet domains. As shown for the recovery of object phase of rotavirus double-layered particles [9] in Fig. 2, this algorithm is robust to the decreased probe overlap ratio due to the subsampling. Moreover, we highlight the importance of the sampling strategy and show that the subsampling patterns generated purely at random are unreliable. We present a pseudo-random sampling of the probe positions to prevent very short and long probe jumps, which in turn reduces issues of scanning coil hysteresis caused by large probe movements and potential beam damage due to densely populated probe positions [10]. For beam sensitive materials, this sampling strategy could help to reduce damage accumulation effects that are mediated by a diffusion process, which can be enhanced when neighbouring positions are scanned [10].  

Figure 1. Schematic of conventional vs. compressive Electron Ptychography. A 2-D sampling strategy controls the subsampling of the probe positions. Our reconstruction algorithm is a regularized version of the EPIE algorithm and leverages, as a prior knowledge, the sparsity of the object phase and amplitude in a transform domain, e.g., Discrete Cosine Transform.

Figure 2. Conventional vs. proposed regularisation of EPIE. Reconstructed object phase of rotavirus particles [9] are shown with estimated probe amplitudes overlaid. Conventional EPIE fails to recover object phase from subsampled data, while our algorithm yields high-quality images of object phase as well as amplitude of the probe.

References

[1] J Rodenburg and A Maiden in “Springer handbook of microscopy”, eds. PW Hawkes and JCH Spence (Springer International Publishing, Cham, Switzerland), pp. 819-904.

[2] A Maiden and J Rodenburg, Ultramicroscopy, 109 (2009), pp. 1256–1262.

[3] P Thibault et al., Ultramicroscopy, 109 (2009), pp. 338–343.

[4] DR Luke, Inverse Problems, 21 (2005), pp. 37–50. 

[5] D Nicholls et al., IEEE International Conference on Acoustics, Speech, and Signal Processing (2022), pp. 1586-1590.

[6] D Nicholls et al., arXiv preprint, arXiv:2211.03494 (2023).

[7] DL Donoho, IEEE Transactions on Information. Theory, 52 (2006), pp. 1289-1306. 

[8] A Stevens et al., Applied Physics Letters, 113 (2018), pp. 033104.

[9] L Zhou et al., Nature Communications, 11 (2020), 2773.

[10] A Velazco et al., Ultramicroscopy, 232 (2022), pp. 113398.